MATHS

The Gambler’s Fallacy and Probability 🎰

Picture yourself in a casino, your palms sweating as the roulette wheel spins. The last 25 balls have landed on black, so you bet ‘all-in’ on red, thinking there must be a better chance of the ball landing on red this time. This is an example of the Gambler’s Fallacy. It's a captivating illusion that tricks us into believing that past events influence future outcomes. This psychological quirk has profound implications, not just in games of chance, but also in our everyday decisions. The fallacy hinges on the misconception that probability adjusts based on previous results, when in fact, each spin of the wheel is independent.

💡 Things to consider

• Conditional Probability and Sequences: Conditional probability explores the likelihood of an event occurring given certain conditions. Consider a coin flip – the probability of getting heads on the second flip doesn't change based on the outcome of the first. To deepen your understanding, explore Bayes' theorem, a powerful tool in assessing conditional probabilities. Bayes’ theorem not only has important implications in the realm of Mathematics, but also in other surprising places, such as the Philosophy of Religion and arguments around the ‘probability’ of God’s existence.

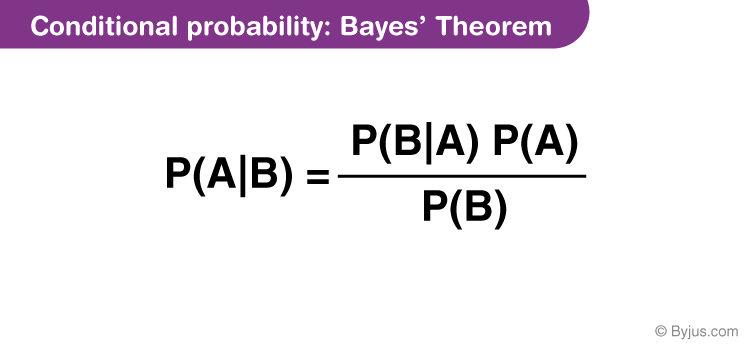
• Law of Large Numbers and Convergence: Uncover the mathematical magic of the Law of Large Numbers. This principle asserts that as you increase the number of trials, the experimental probability approaches the theoretical probability. This mathematical truth dismantles the fallacy's illusion of predictability. To deepen your exploration, delve into the Central Limit Theorem, which sheds light on the distribution of outcomes in large trials.

• Real-Life Ramifications: Extend your exploration beyond the casino floor. Investigate cases where the fallacy's influence has had significant real-life consequences, from financial misjudgements to superstitious behaviours. One particularly interesting one to look out for is the anxiety surrounding childbirth in previous years. Pierre-Simon Laplace in A Philosophical Essay on Probabilities described some of these cases where fathers were worried that the probability of their child being a daughter would increase if everyone in their community is having a son.

🔎 Find out more

• Gambler's Fallacy: Overview and Examples

• Law of Large Numbers (YouTube)

🍒 The cherry on top

• 🐉 Orientalism: The article explores the life and work of Robert Irwin, a scholar of the medieval Near East who was also a Sufi mystic. Irwin's defence of Orientalism, a field of study that has been criticised for its Eurocentric perspective, was controversial. But Irwin believed that Orientalism could be a valuable tool for understanding the cultures of the Middle East. This is great if you’re into History, Literature, or Art.

• ➕ Gottlob Frege: Gottlob Frege was one of the most influential philosophers of mathematics, logic and language. The developments he instigated in those fields continue to define them, especially in the English speaking world. Learn more about him in this article if you’re into Philosophy or Maths.

• 🌳 Trees holding their breath: When wildfire smoke is in the air, doctors urge people to stay indoors to avoid breathing in harmful particles and gases. But what happens to trees and other plants that can’t escape from the smoke? Check out this article if you’re into Biology.

👀 Keep your eyes peeled for…

• 5th August:

  • King’s College Cambridge Personal Statement Webinar

• 6th August:

  • King’s College Cambridge Intro to Cambridge Webinar

• 7th August:

  • University of Buckingham Computing Taster Webinar

• 8th August:

  • UEA Managing Results Day Uncertainty Webinar

🗳️ Poll